Membrane potential

Differences in concentration of ions on opposite sides of a cellular membrane produce a voltage difference called the membrane potential. The largest contributions usually come from sodium (Na⁺) and chloride (Cl^–) ions which have high concentrations in the extracellular region, and potassium (K⁺) ions, which along with large protein anions have high concentrations in the intracellular region. Calcium ions, which sometimes play an important role, are not shown.

Membrane potential (or transmembrane potential) is the difference in voltage (or electrical potential difference) between the interior and exterior of a cell $(V_{interior}-V_{exterior})$ . All animal cells are surrounded by a plasma membrane composed of a lipid bilayer with many diverse protein assemblages embedded in it. The fluid on both sides of the membrane contains high concentrations of mobile ions, of which sodium (Na⁺), potassium (K⁺), chloride (Cl^–), and calcium (Ca²⁺) are the most important. The membrane potential arises from the interaction of ion channels and ion pumps embedded in the membrane, which maintain different ion concentrations on the intracellular and extracellular sides of the membrane.

The membrane potential has two basic functions. First, it allows a cell to function as a battery, providing power to operate a variety of "molecular devices" embedded in the membrane. Second, in electrically excitable cells such as neurons, it is used for transmitting signals between different parts of a cell. Opening or closing of ion channels at one point in the membrane produces a local change in the membrane potential, which causes electric current to flow rapidly to other points in the membrane.

In non-excitable cells, and in excitable cells in their baseline states, the membrane potential is held at a relatively stable value, called the resting potential. For neurons, typical values of the resting potential range from -70 to -80 millivolts; that is, the interior of a cell has a negative baseline voltage of a bit less than one tenth of a volt. Opening and closing of ion channels can induce a departure from the resting potential, called a depolarization if the interior voltage rises (say from -70 mV to -65 mV), or a hyperpolarization if the interior voltage becomes more negative (changing from -70 mV to -80 mV, for example). In excitable cells, a sufficiently large depolarization can evoke a short-lasting all-or-nothing event called an action potential, in which the membrane potential very rapidly undergoes a large change, often briefly reversing its sign. Action potentials are generated by special types of voltage-dependent ion channels.

In neurons, the factors that influence the membrane potential are diverse. They include numerous types of ion channels, some that are chemically gated and some that are voltage-gated. Because voltage-dependent ion channels are controlled by the membrane potential, while the membrane potential itself is partly controlled by these same ion channels, feedback loops arise which allow for complex temporal dynamics, including oscillations and regenerative events such as action potentials.

Physical basis

The membrane potential in a cell derives ultimately from two factors: electrical force and diffusion. Electrical force arises from the mutual attraction between particles with opposite electrical charges (positive and negative) and the mutual repulsion between particles with the same type of charge (both positive or both negative). Diffusion arises from the statistical tendency of particles to redistribute from regions where they are highly concentrated to regions where the concentration is low.

Voltage

Electric field (arrows) and contours of constant voltage created by a pair of oppositely-charged objects. The electric field is at right angles to the voltage contours, and the field is strongest where the spacing between contours is the smallest.

Voltage, which is synonymous with electrical potential, is relatively simple to define mathematically, but not easy to explain concretely in a non-mathematical way. Intuitively, voltage is the ability to drive an electrical current. If a voltage source such as a battery is placed in an electrical circuit, the higher the voltage of the source, the greater the amount of current that it will drive. In a functioning circuit, each point can be assigned a voltage level—the voltage difference between any two points determines the amount of current that would flow through a wire hooked directly from one point to the other. In practical electronics, the voltage difference between two points can be measured by connecting them to the two leads of a volt meter (voltmeter).

The functional significance of voltage lies only in voltage differences—the absolute value of voltage has no significance. A volt meter can measure the voltage difference between two locations in a circuit, but there is no instrument that can measure the voltage at a single point: the concept has no meaning. It is conventional in electronics to assign a voltage of zero to some arbitrarily chosen element of the circuit, and then assign voltages for other elements on the basis of the measured or calculated voltage differences, but there is no significance in which element is chosen as the zero point—the function of a circuit depends only on the differences, not on voltages per se.

The same principle applies to voltage in cell biology. In electrically active tissue, the voltage difference between any two points can be measured by inserting an electrode at each point and connecting both electrodes to the leads of a volt meter. There is no way, however, to measure the voltage of a single point. Thus, a statement that the voltage difference across the membrane of a cell is 60 millivolts can be verified by placing electrodes inside and outside the cell—but whether the exterior is assigned a voltage of 60 mV and the interior 0 mV, or the exterior is assigned a voltage of 0 mV and the interior -60 mV, has no significance; only the difference between the two matters, not the absolute number assigned to either.

In mathematical terms, the definition of voltage begins with the concept of an electric field $E$ , a vector field assigning a magnitude and direction to each point in space. In many situations, the electric field is a conservative field, which means that it can be expressed as the gradient of a scalar function $V$ , that is, $E = \nabla V$ . This scalar field $V$ is referred to as the voltage distribution. Note that the definition allows for an arbitrary constant of integration—this is why absolute values of voltage are not meaningful. In general electric fields can only be treated as conservative if magnetic fields do not significantly influence them, but this condition usually applies well to biological tissue.

Because the electric field is the gradient of the voltage distribution, rapid changes in voltage within a small region imply a strong electric field; conversely, if the voltage remains approximately the same over a large region, the electric fields in that region must be weak. A strong electric field, equivalent to a strong voltage gradient, implies that a strong force is exerted on any charged particles that lie within the region.

Salts and ions in an aqueous medium

The fluid both inside and outside of animal cells (intracellular and extracellular) contains a high concentration of dissolved salts. When salts dissolve in water, they break apart into ions—for example sodium chloride (NaCl) breaks up almost entirely into positively charged sodium ions (Na⁺) and negatively charged chloride (Cl^–) ions. Small ions such as sodium (Na⁺), potassium (K⁺), calcium (Ca⁺⁺), and chloride (Cl^–) are present in high concentrations, and are capable of diffusing freely from place to place, unless some type of barrier impedes them.

Plasma membrane

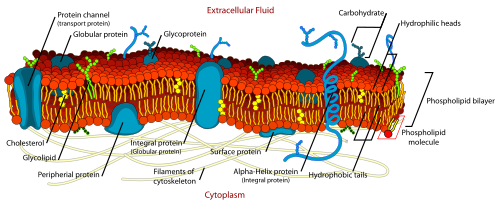
The cell membrane, also called the plasma membrane or plasmalemma, is a semipermeable lipid bilayer common to all living cells. It contains a variety of biological molecules, primarily proteins and lipids, which are involved in a vast array of cellular processes.

Every animal cell is enclosed in a plasma membrane, which has the structure of a lipid bilayer with many types of large molecules embedded in it. Because it is made of lipid molecules, the plasma membrane intrinsically has a high electrical resistivity, in other words a low intrinsic permeability to ions. However, some of the molecules embedded in the membrane are capable either of actively transporting ions from one side of the membrane to the other, or of providing channels through which they can move.

In electrical terminology, the plasma membrane functions as a combined resistor and capacitor. Resistance arises from the fact that the membrane impedes the movement of charges across it. Capacitance arises from the fact that the lipid bilayer is so thin that an accumulation of charged particles on one side gives rise to an electrical force that pulls oppositely-charged particles toward the other side. The capacitance of the membrane is relatively unaffected by the molecules that are embedded in it, so it has a more or less invariant value estimated at about 2 µF/cm² (the total capacitance of a patch of membrane is proportional to its area). The conductance of a pure lipid bilayer is so low, on the other hand, that in biological situations it is always dominated by the conductance of alternative pathways provided by embedded molecules. Thus the capacitance of the membrane is more or less fixed, but the resistance is highly variable.

The thickness of a plasma membrane is estimated to be about 7-8 nanometers. Because the membrane is so thin, it does not take a very large transmembrane voltage to create a strong electric field within it. Typical membrane potentials in animal cells are on the order of 100 millivolts (that is, one tenth of a volt), but calculations show that this generates an electric field close to the maximum that the membrane can sustain—it has been calculated that a voltage difference much larger than 200 millivolts could cause dielectric breakdown, that is, arcing across the membrane.

Facilitated diffusion and transport

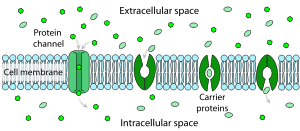
Facilitated diffusion in cell membranes, showing ion channels and carrier proteins

The resistance of a pure lipid bilayer to the passage of ions across it is very high, but structures embedded in the membrane can greatly enhance ion movement, either actively or passively, via mechanisms called facilitated transport and facilitated diffusion. The two types of structure that play the largest roles are ion channels and ion pumps, both usually formed from assemblages of protein molecules. Ion channels provide passageways through which ions can move. In most cases an ion channel is only permeable to specific types of ions (for example sodium and potassium but not chloride or calcium), and sometimes the permeability varies depending on the direction of ion movement. Ion pumps, also known as ion transporters or carrier proteins, actively transport specific types of ions from one side of the membrane to the other, sometimes using energy derived from metabolic processes to do so.

Ion pumps

The sodium-potassium pump uses energy derived from ATP to exchange sodium for potassium ions across the membrane.

A major contribution to establishing the membrane potential is made by the sodium-potassium exchange pump. This is a complex of proteins embedded in the membrane that derives energy from ATP in order to transport sodium and potassium ions across the membrane. On each cycle, the pump exchanges three Na⁺ ions from the intracellular space for two K⁺ ions from the extracellular space. If the numbers of each type of ion were equal, the pump would be electrically neutral, but because of the three-for-two exchange, it gives a net movement of one positive charge from intracellular to extracellular for each cycle, thereby contributing to a positive voltage difference. The pump has three effects: (1) it makes the sodium concentration high in the extracellular space and low in the intracellular space; (2) it makes the potassium concentration high in the intracellular space and low in the extracellular space; (3) it gives the extracellular space a positive voltage with respect to the intracellular space.

The sodium-potassium exchange pump is relatively slow in operation. If a cell were initialized with equal concentrations of sodium and potassium everywhere, it would take hours for the pump to establish equilibrium. The pump operates constantly, but becomes progressively less efficient as the concentrations of sodium and potassium available for pumping are reduced.

Another functionally important ion pump is the sodium-calcium exchanger. This pump operates in a conceptually similar way to the sodium-potassium pump, except that in each cycle it exchanges three Na⁺ from the extracellular space for one Ca⁺⁺ from the intracellular space. Because the net flow of charge is inward, this pump runs "downhill", effectively, and therefore does not require any energy source except the membrane voltage. Its most important effect is to pump calcium outward—it also allows an inward flow of sodium, thereby counteracting the sodium-potassium pump, but because overall sodium and potassium concentrations are much higher than calcium concentrations, this effect is relatively unimportant. The net result of the sodium-calcium exchanger is that in the resting state, intracellular calcium concentrations become very low.

Ion channels

As explained above, a pure lipid bilayer has a very low permeability to ions of any type. However, animal cell membranes contain a very diverse set of ion channels, which are protein structures embedded in the membrane that allow passage of specific types of ions under specific conditions. These can be divided into three types: leakage channels, ligand-gated channels, and voltage-dependent channels. This categorization is not exhaustive—it leaves out sensory receptors, many of which depend on ion channels that are activated by physical stimuli such as light, temperature, or stretching.

Leakage channels

Leakage channels are the simplest type, in that their permeability is more or less constant. The types of leakage channels that have the greatest significance in neurons are potassium and chloride channels. It should be noted that even these are not perfectly constant in their properties: first, most of them are voltage-dependent in the sense that they conduct better in one direction than the other (in other words, they are rectifiers); second, some of them are capable of being shut off by chemical ligands even though they do not require ligands in order to operate.

Ligand-gated channels

Ligand-gated calcium channel in closed and open states

Ligand-gated ion channels are channels whose permeability is greatly increased when some type of chemical ligand binds to the protein structure. Animal cells contain hundreds, if not thousands, of types of these. A large subset function as neurotransmitter receptors—they occur at postsynaptic sites, and the chemical ligand that gates them is released by the presynaptic axon terminal. One example of this type is the AMPA receptor, a receptor for the neurotransmitter glutamate that when activated allows passage of sodium and potassium ions. Another example is the GABA_A receptor, a receptor for the neurotransmitter GABA that when activated allows passage of chloride ions.

Neurotransmitter receptors are activated by ligands that appear in the extracellular area, but there are other types of ligand-gated channels that are controlled by interactions on the intracellular side.

Voltage-dependent channels

Voltage-gated ion channels, also known as voltage dependent, are channels whose permeability is influenced by the membrane potential. They form another very large group, with each member having a particular ion selectivity and a particular voltage dependence. Many are also time-dependent—in other words, they do not respond immediately to a voltage change, but only after a delay.

One of the most important members of this group is a type of voltage-gated sodium channel that underlies action potentials—these are sometimes called Hodgkin-Huxley sodium channels because they were initially characterized by Alan Lloyd Hodgkin and Andrew Huxley in their Nobel Prize-winning studies of the physiology of the action potential. The channel is closed at the resting voltage level, but opens abruptly when the voltage exceeds a certain threshold, allowing a large influx of sodium ions that produces a very rapid change in the membrane potential. Recovery from an action potential is partly dependent on a type of voltage-gated potassium channel which is closed at the resting voltage level but opens as a consequence of the large voltage change produced during the action potential.

Some voltage-dependent ion channels are also at the same time ligand-gated. One of the best known of these is the NMDA receptor, a type of calcium channel that is gated by the neurotransmitter glutamate but also requires the membrane potential to be elevated substantially above baseline in order to open.

Reversal potential

The reversal potential (or equilibrium potential) of an ion is the value of transmembrane voltage at which diffusive and electrical forces counterbalance, so that there is no net ion flow across the membrane. This means that the transmembrane voltage exactly opposes the force of diffusion of the ion , such that the net current of the ion across the membrane is zero and unchanging. The reversal potential is important because it gives the voltage that acts on channels permeable to that ion—in other words, it gives the voltage that the ion concentration gradient generates when it acts as a battery.

The equilibrium potential of a particular ion is usually designated by the notation E_ion.The equilibrium potential for any ion can be calculated using the Nernst equation.^[1] For example, reversal potential for potassium ions will be as follows:

$E_{eq,K^+} = \frac{RT}{zF} \ln \frac{[K^+]_{o}}{[K^+]_{i}} ,$

where

E_eq,K⁺ is the equilibrium potential for potassium, measured in volts
R is the universal gas constant, equal to 8.314 joules·K⁻¹·mol⁻¹
T is the absolute temperature, measured in kelvins (= K = degrees Celsius + 273.15)
z is the number of elementary charges of the ion in question involved in the reaction
F is the Faraday constant, equal to 96,485 coulombs·mol⁻¹ or J·V⁻¹·mol⁻¹
[K⁺]_o is the extracellular concentration of potassium, measured in mol·m⁻³ or mmol·l⁻¹
[K⁺]_i is the intracellular concentration of potassium

Even if two different ions have the same charge (i.e. K⁺ and Na⁺), they can still have very different equilibrium potentials, provided their outside and/or inside concentrations differ. Take, for example, the equilibrium potentials of potassium and sodium in neurons. The potassium equilibrium potential E_K is -84 mV with 5 mM potassium outside and 140 mM inside. The sodium equilibrium potential, on the other hand, E_Na is approximately +40 mV with approximately 12 mM sodium inside and 140 mM outside.^{[note 1]}

Equivalent circuit

Equivalent circuit for a patch of membrane, consisting of a fixed capacitance in parallel with four pathways each containing a battery in series with a variable conductance

Electrophysiologists model the effects of ionic concentration differences, ion channels, and membrane capacitance in terms of an equivalent circuit, which is intended to represent the electrical properties of a small patch of membrane. The equivalent circuit consists of a capacitor in parallel with four pathways each consisting of a battery in series with a variable conductance. The capacitance is determined by the properties of the lipid bilayer, and is taken to be fixed. Each of the four parallel pathways comes from one of the principal ions, sodium, potassium, chloride, and calcium. The voltage of each ionic pathway is determined by the concentrations of the ion on each side of the membrane; see the Reversal potential section below. The conductance of each ionic pathway at any point in time is determined by the states of all the ion channels that are potentially permeable to that ion, including leakage channels, ligand-gated channels, and voltage-dependent channels.

Reduced circuit obtained by combining the ion-specific pathways using the Goldman equation

For fixed ion concentrations and fixed values of ion channel conductance, the equivalent circuit can be further reduced, using the Goldman equation as described below, to a circuit containing a capacitance in parallel with a battery and conductance. Electrically this is a type of RC circuit (resistance-capacitance circuit), and its electrical properties are very simple. Starting from any initial state, the current flowing across either the conductance or capacitance decays with an exponential time course, with a time constant of $τ = RC$ , where $C$ is the capacitance of the membrane patch, and $R = 1/g net$ is the net resistance. For realistic situations the time constant usually lies in the 1—100 millisecond range. In most cases changes in the conductance of ion channels occur on a faster time scale, so an RC circuit is not a good approximation; however the differential commonly equation used to model a membrane patch is a modified version of the RC circuit equation.

Resting potential

When the membrane potential of a cell can go for a long period of time without changing significantly, it is referred to as a resting potential or resting voltage. This term is used for the membrane potential of non-excitable cells, but also for the membrane potential of excitable cells in the absence of excitation. In excitable cells, the other possible states are graded membrane potentials (of variable amplitude), and action potentials, which are large, all-or-nothing rises in membrane potential that usually follow a fixed time course. Excitable cells include neurons, muscle cells, and some secretory cells in glands. Even in other types of cells, though, the membrane voltage can undergo changes in response to environmental or intracellular stimuli. For example, depolarization of the plasma membrane appears to be an important step in programmed cell death.^[2]

The interactions that generate the resting potential are modeled by the Goldman equation.^[3] This is similar in form to the Nernst equation shown above, in that it is based on the charges of the ions in question, as well as the difference between their inside and outside concentrations. However, it also takes into consideration the relative permeability of the plasma membrane to each ion in question.

$E_{m} = \frac{RT}{F} \ln{ \left( \frac{ P_{\mathrm{K}}[\mathrm{K}^{+}]_\mathrm{out} + P_{\mathrm{Na}}[\mathrm{Na}^{+}]_\mathrm{out} + P_{\mathrm{Cl}}[\mathrm{Cl}^{-}]_\mathrm{in}}{ P_{\mathrm{K}}[\mathrm{K}^{+}]_\mathrm{in} + P_{\mathrm{Na}}[\mathrm{Na}^{+}]_\mathrm{in} + P_{\mathrm{Cl}}[\mathrm{Cl}^{-}]_\mathrm{out}} \right) }$

The three ions that appear in this equation are potassium (K⁺), sodium (Na⁺), and chloride (Cl⁻). Calcium is omitted, but can be added to deal with situations in which it plays a significant role.^[4] Being an anion, the chloride terms are treated differently than the cation terms; the intracellular concentration is in the numerator, and the extracellular concentration in the denominator, which is reversed from the cation terms. P_i stands for the relative permeability of the ion type i.

The Goldman formula essentially expresses the membrane potential as a weighted average of the reversal potentials for the individual ion types, weighted by permeability. In most animal cells, the permeability to potassium is much higher in the resting state than the permeability to sodium. Consequently, the resting potential is usually close to the potassium reversal potential.^[5]^[6] The permeability to chloride can be high enough to be significant, but unlike the other ions, chloride is not actively pumped, and therefore equilibrates at a reversal potential very close to the resting potential determined by the other ions.

Values of resting membrane potential in the most animal cells usually vary between the potassium reversal potential (usually around -80 mV) and around -40 mV. The resting potential in excitable cells (capable of producing action potentials) is usually near -60 mV—more depolarized voltages would lead to spontaneous generation of action potentials. Immature or undifferentiated cells show highly variable values of resting voltage, usually significantly more positive than in differentiated cells.^[7] In such cells, the resting potential value correlates with the degree of differentiation: undifferentiated cells in some cases may not show any transmembrane voltage difference at all.

Maintenance of the resting potential can be metabolically costly for a cell because of its requirement for active pumping of ions to counteract losses due to leakage channels. The cost is highest when the cell function requires an especially depolarized value of membrane voltage. For example, the resting potential in daylight-adapted blowfly (Calliphora vicina) photoreceptors can be as high as -30 mV.^[8]. This elevated membrane potential allows the cells to respond very rapidly to visual inputs; the cost is that maintenance of the resting potential may consume more than 20% of overall cellular ATP.^[9]

On the other hand, the high resting potential in undifferentiated cells can be a metabolic advantage. This apparent paradox is resolved by examination of the origin of that resting potential. Little-differentiated cells are characterized by extremely high input resistance^[7] which implies that few leakage channels are present at this stage of cell life. As an apparent result, potassium permeability becomes similar to that for sodium ions, which places resting potential in-between the reversal potentials for sodium and potassium as discussed above. The reduced leakage currents also mean there is little need for active pumping in order to compensate, therefore low metabolic cost.

Graded potentials

As explained above, the membrane potential at any point in a cell's membrane is determined by the ion concentration differences between the intracellular and extracellular areas, and by the permeability of the membrane to each type of ion. The ion concentrations do not normally change very quickly (with the exception of calcium, where the baseline intracellular concentration is so low that even a small inflow may increase it by orders of magnitude), but the permeabilities can change in a fraction of a millisecond, as a result of activation of ligand-gated or voltage-gated ion channels. The change in membrane potential can be large or small, depending on how many ion channels are activated and what type they are. Changes of this type are referred to as graded potentials, in contrast to action potentials, which have a fixed amplitude and time course.

As can be derived from the Goldman equation shown above, the effect of increasing the permeability for a particular type of ion is to shift the membrane potential toward the reversal potential for that ion. Thus, opening sodium channels pulls the membrane potential toward the sodium reversal potential, usually around +100 mV. Opening potassium channels pulls the membrane potential toward about -90 mV; opening chloride channels pulls it toward about -70 mV. Because -90 to +100 mV is the full operating range of membrane potential, the effect is that sodium channels always pull the membrane potential up, potassium channels pull it down, and chloride channels pull it toward the resting potential.

Graph displaying an EPSP, an IPSP, and the summation of an EPSP and an IPSP.

Graded membrane potentials are particularly important in neurons, where they are produced by synapses—a temporary rise or fall in membrane potential produced by activation of a synapse is called a postsynaptic potential. Neurotransmitters that act to open sodium channels cause the membrane potential to rise, while neurotransmitters that act on potassium channels cause it to fall. Because the membrane potential in a neuron must rise past the threshold value to produce an action potential, a rise in membrane potential is excitatory, while a fall is inhibitory. Thus neurotransmitters that act to open sodium channels produce a so-called excitatory postsynaptic potential, or EPSP, whereas neurotransmitters that act to open potassium channels produce an inhibitory postsynaptic potential, or IPSP. When multiple types of channels are open within the same time period, their postsynaptic potentials summate.

All other values of membrane potential

From the viewpoint of biophysics, there is nothing particularly special about the resting membrane potential. It is merely the membrane potential that results from the membrane permeabilities that predominate when the cell is resting. The above equation of weighted averages always applies, but the following approach may be easier to visualize. At any given moment, there are two factors for an ion that determine how much influence that ion will have over the membrane potential of a cell.

That ion's driving force and,
That ion's permeability

Intuitively, this is easy to understand. If the driving force is high, then the ion is being "pushed" across the membrane hard (more correctly stated: it is diffusing in one direction faster than the other). If the permeability is high, it will be easier for the ion to diffuse across the membrane. But what are 'driving force' and 'permeability'?

Driving force: the driving force is the net electrical force available to move that ion across the membrane. It is calculated as the difference between the voltage that the ion "wants" to be at (its equilibrium potential) and the actual membrane potential (E_m). So formally, the driving force for an ion = E_m - E_ion

For example, at our earlier calculated resting potential of −73 mV, the driving force on potassium is 7 mV (−73 mV) − (−80 mV) = 7 mV. The driving force on sodium would be (−73 mV) − (60 mV) = −133 mV.

Permeability: is simply a measure of how easily an ion can cross the membrane. It is normally measured as the (electrical) conductance and the unit, siemens, corresponds to 1 C·s⁻¹·V⁻¹, that is one charge per second per volt of potential.

So in a resting membrane, while the driving force for potassium is low, its permeability is very high. Sodium has a huge driving force, but almost no resting permeability. In this case, the math tells us that potassium carries about 20 times more current than sodium, and thus has 20 times more influence over E_m than does sodium.

However, consider another case—the peak of the action potential. Here permeability to Na is high and K permeability is relatively low. Thus the membrane moves to near E_Na and far from E_K.

The more ions are permeant, the more complicated it becomes to predict the membrane potential. However, this can be done using the Goldman-Hodgkin-Katz equation or the weighted means equation. By simply plugging in the concentration gradients and the permeabilities of the ions at any instant in time, one can determine the membrane potential at that moment. What the GHK equations says, basically, is that at any time, the value of the membrane potential will be a weighted average of the equilibrium potentials of all permeant ions. The "weighting" is the ions relative permeability across the membrane.

Effects and implications

While cells expend energy to transport ions and establish a transmembrane potential, they use this potential in turn to transport other ions and metabolites such as sugar. The transmembrane potential of the mitochondria drives the production of ATP, which is the common currency of biological energy.

Cells may draw on the energy they store in the resting potential to drive action potentials or other forms of excitation. These changes in the membrane potential enable communication with other cells (as with action potentials) or initiate changes inside the cell, which happens in an egg when it is fertilized by a sperm.

In neuronal cells, an action potential begins with a rush of sodium ions into the cell through sodium channels, resulting in depolarization, while recovery involves an outward rush of potassium through potassium channels. Both these fluxes occur by passive diffusion.

Notes

↑ Note that the sign of E_Na and E_K are opposite. This is because the concentration gradient for potassium is directed out of the cell, while the concentration gradient for sodium is directed into the cell. Membrane potentials are defined relative to the exterior of the cell; thus, a potential of −70 mV implies that the interior of the cell is negative relative to the exterior.

References

↑ Purves et al., pp. 28–32; Bullock, Orkand, and Grinnell, pp. 133–134; Schmidt-Nielsen, pp. 478–480, 596–597; Junge, pp. 33–35
↑ Franco R, Bortner CD, Cidlowski JA (January 2006). "Potential roles of electrogenic ion transport and plasma membrane depolarization in apoptosis". J. Membr. Biol. 209 (1): 43–58. doi:10.1007/s00232-005-0837-5. PMID 16685600.
↑ Purves et al., pp. 32–33; Bullock, Orkand, and Grinnell, pp. 138–140; Schmidt-Nielsen, pp. 480; Junge, pp. 35–37
↑ Spangler SG (1972). "Expansion of the constant field equation to include both divalent and monovalent ions". Ala J Med Sci 9 (2): 218–23. PMID 5045041.
↑ Purves et al., p. 34; Bullock, Orkand, and Grinnell, p. 134; Schmidt-Nielsen, pp. 478–480.
↑ Purves et al., pp. 33–36; Bullock, Orkand, and Grinnell, p. 131.
↑ ^7.0 ^7.1 Magnuson DS, Morassutti DJ, Staines WA, McBurney MW, Marshall KC. (1995 Jan 14). "In vivo electrophysiological maturation of neurons derived from a multipotent precursor (embryonal carcinoma) cell line". Brain Res Dev Brain Res. 84 (1): 130–41. doi:10.1016/0165-3806(94)00166-W. PMID 7720212.
↑ Juusola M, Kouvalainen E, Järvilehto M, Weckström M. (1994 Sep). "Contrast gain, signal-to-noise ratio, and linearity in light-adapted blowfly photoreceptors". J Gen Physiol. 104 (3): 593–621. doi:10.1085/jgp.104.3.593. PMID 7807062.
↑ Laughlin SB, de Ruyter van Steveninck RR, Anderson JC (1998 May). "The metabolic cost of neural information". Nat Neurosci. 1 (1): 36–41. doi:10.1038/236. PMID 10195106.

External links

Nernst/Goldman Equation Simulator